Radiation pressure
Radiation pressure is the pressure exerted upon any surface exposed to electromagnetic radiation. If absorbed, the pressure is the power flux density divided by the speed of light. If the radiation is totally reflected, the radiation pressure is doubled. For example, the radiation of the Sun at the Earth has a power flux density of 1,370 W/m2, so the radiation pressure is 4.6 µPa (absorbed).
Contents |
Discovery
The fact that electromagnetic radiation exerts a pressure upon any surface exposed to it was deduced theoretically by James Clerk Maxwell in 1871 and Adolfo Bartoli in 1876, and proven experimentally by Russian physicist Peter Lebedev in 1900[1] and by Ernest Fox Nichols and Gordon Ferrie Hull in 1901.[2] The pressure is very feeble, but can be detected by allowing the radiation to fall upon a delicately poised vane of reflective metal in a Nichols radiometer (this should not be confused with the Crookes radiometer, whose characteristic motion is not caused by radiation pressure but by impacting gas molecules).
Theory
It may be shown by electromagnetic theory, by quantum theory, or by thermodynamics, making no assumptions as to the nature of the radiation, that the pressure against a surface exposed in a space traversed by radiation uniformly in all directions is equal to one third of the total radiant energy per unit volume within that space.
Quantum Theory Argument
From the perspective of quantum theory, light is made of photons: particles with zero mass but which carry energy and - importantly in this argument - momentum . According to special relativity, because photons are massless their energy (E) and momentum (p) are related by E=pc [3].
Now consider a beam of light perpendicularly incident on a surface, and let us assume the beam of light is totally absorbed. If we imagine the beam is made of photons, then every second numerous photons strike the surface and are absorbed. The momentum the photons carry is a conserved quantity - it cannot be destroyed - so it must be transferred to the surface, the result is that absorbing the light beam causes the surface to gain momentum.
Newton's Second Law tells us that force equals rate of change of momentum, so during each second the surface experiences a force (or pressure, as pressure is force per unit area) due to the momentum the photons transfer to it. We have:
Pressure = momentum transferred per second per unit area = Energy deposited per second per unit area / c = I/c.
Where I is the intensity of the beam of light (measured in e.g. W⋅m-2).
In the above argument we assumed that the surface totally absorbed the beam, in general light can be transmitted, reflected and/or absorbed. If the light were totally reflected then the radiation pressure is doubled compared to total absorption, this is because the photons arrive with momentum E/c and depart with momentum -E/c (the -ve sign indicates traveling in the opposite direction), so the change of momentum is 2E/c.
In interplanetary space
In astronomy, Solar radiation pressure is the force exerted by solar radiation on objects within its reach. Solar radiation pressure is of interest in astrodynamics, as it is one source of the orbital perturbations.
The disturbance force can be expressed simply as
where:
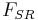 - Force contributed by the solar radiation pressure
- Force contributed by the solar radiation pressure
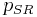 - Force per unit area exerted by the solar radiation
- Force per unit area exerted by the solar radiation
 - Coefficient of reflectivity of the object
- Coefficient of reflectivity of the object
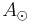 - Area of the object exposed to the solar radiation
- Area of the object exposed to the solar radiation
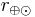 - radial unit vector between object and the Sun
- radial unit vector between object and the Sun
Radiation pressure is about 10−5 Pa at Earth's distance from the Sun[5] and decreases by the square of the distance from the Sun.
For example, at the boiling point of water (T = 373 K), a blackbody is emitting about 1,080 watts of energy per square meter of surface. This is somewhat below the Sun's 1373 W/m², but still instructive. If the blackbody absorbs 1,080 watts on its sun-facing surface, it must also emit all 1,080 watts omnidirectionally. The omnidirectional emission is self-cancelling, so that it neither adds nor detracts from the net solar flux force.
By the radiation pressure equation σT4/c; the sun-facing photon pressure is 3.61 µPa (3.6 N/km², 2.08 lbf/mi²). If the sun-facing surface is an almost perfect reflector, the force would approach double that (7.22 µPa) depending on how close to an ideal reflector the surface is polished.
While rather small in comparison to chemical thrusters, the radiation pressure force is inexorable and requires no fuel mass. Thus over months-to-years, the net (integrated) amount of force is substantial, and is thought to be sufficient to speed interplanetary probes to velocities that could traverse the Earth-Pluto distance in 1/2 to 1/4 the time of a chemically accelerated vessel.
Such feeble pressures are able to produce marked effects upon minute particles like gas ions and electrons, and are important in the theory of electron emission from the Sun, of cometary material, and so on (see also: Yarkovsky effect, YORP effect, Poynting–Robertson effect).
| AU distance | µPa (µN/m²) | N/km² | lbf/mi² |
|---|---|---|---|
| 0.10 AU = Close | 915 | 915 | 526 |
| 0.46 AU = Mercury | 43.3 | 43.3 | 24.9 |
| 0.72 AU = Venus | 17.7 | 17.7 | 10.2 |
| 1.00 AU = Earth | 9.15 | 9.15 | 5.26 |
| 1.52 AU = Mars | 3.96 | 3.96 | 2.28 |
| 5.22 AU = Jupiter | 0.34 | 0.34 | 0.19 |
The table shows that the accelerative forces very close to the Sun are very high, and almost of no comparative importance (for macroscopic particles) by the orbital distance of Jupiter. It is for this reason that most interplanetary radiation-pressure probe missions are sun grazers, whose orbital trajectory passes very close to the Sun so that at midpoint, the probe's reflectors can be turned toward the Sun, adding considerable velocity to the craft.
Because the ratio of surface area to volume (and thus mass) increases with decreasing particle size, dusty (micrometre-size) particles are susceptible to radiation pressure even in the outer solar system. For example, the evolution of the outer rings of Saturn is significantly influenced by radiation pressure.
Poynting vector and radiation pressure
S divided by the square of the speed of light in free space is the density of the linear momentum of the electromagnetic field. The time-averaged intensity  divided by the speed of light in free space is the radiation pressure exerted by an electromagnetic wave on the surface of a target:
divided by the speed of light in free space is the radiation pressure exerted by an electromagnetic wave on the surface of a target:
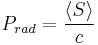 .
.
In stellar interiors
In stellar interiors the temperatures are very high. Stellar models predict a temperature of 15 MK in the center of the Sun and at the cores of supergiant stars the temperature may exceed 1 GK. As the radiation pressure scales as the fourth power of the temperature, it becomes important at these high temperatures. In the Sun, radiation pressure is still quite small when compared to the gas pressure. In the heaviest stars, radiation pressure is the dominant pressure component.[6]
Solar sails
Solar sails, a proposed method of spacecraft propulsion, would use radiation pressure from the Sun as a motive force. Private spacecraft Cosmos 1 was to have used this form of propulsion. The idea was proposed as early as 1924 by Soviet scientist Friedrich Zander.
The Japan Aerospace Exploration Agency (JAXA) has successfully unfurled a solar sail in space which has already succeeded in propelling its payload with the IKAROS project.
Effect on GPS satellites
Variability in solar radiation pressure constitutes the single largest source of error in modelling the orbital dynamics of GPS satellites [7].
Radiation pressure in acoustics
In acoustics, radiation pressure is the unidirectional pressure force exerted at an interface between two media due to the passage of a sound wave. If sound is absorbed in the volume during propagation, a body radiation force builds up. In a fluid, this force generates acoustic streaming.
Laser cooling
Laser cooling is applied to cooling materials very close to absolute zero. Atoms traveling towards a laser light source perceive a doppler effect tuned to the absorption frequency of the target element. The radiation pressure on the atom slows movement in a particular direction until the Doppler effect moves out of the frequency range of the element, causing an overall cooling effect.
References
- ^ P. Lebedev, 1901, "Untersuchungen über die Druckkräfte des Lichtes", Annalen der Physik, 1901
- ^ Nichols, E.F & Hull, G.F. (1903) The Pressure due to Radiation, The Astrophysical Journal,Vol.17 No.5, p.315-351
- ^ See discussion and references in photon
- ^ David A. Vallado, 2007, "Fundamentals of Astrodynamics and Applications," 3rd ed., Microcosm Press, Hawthorne, CA, sec. 8.6.4
- ^ Solar Sail
- ^ Dale A. Ostlie and Bradley W. Carroll, An Introduction to Modern Astrophysics (2nd edition), page 341, Pearson, San Francisco, CA 2007
- ^ IPN Progess Report 42-159 (2004)
Further reading
- Dion, J. L.; Malutta, A.; Cielo, P., "Ultrasonic inspection of fiber suspensions", The Journal of the Acoustical Society of America, Volume 72, Issue 5, November 1982, pp.1524-1526
- F.G. Mitri, "Theoretical calculation of the acoustic radiation force acting on elastic and viscoelastic cylinders placed in a plane standing or quasistanding wave field", The European Physical Journal B - Condensed Matter and Complex Systems, Volume 44, Issue 1, March 2005, Pages 71-78. [1]
- F.G. Mitri, "Theoretical and experimental determination of the acoustic radiation force acting on an elastic cylinder in a plane progressive wave—far-field derivation approach", New Journal of Physics, Volume 8, August 2006, art. no. 138. [2]
- F.G. Mitri, "Calculation of the acoustic radiation force on coated spherical shells in progressive and standing plane waves", Ultrasonics, Volume 44, Issue 3, July 2006, Pages 244-258. [3]
- F.G. Mitri, "Acoustic radiation force on a sphere in standing and quasi-standing zero-order Bessel beam tweezers", Annals of Physics, Volume 323, Issue 7, July 2008, Pages 1604-1620. [4]